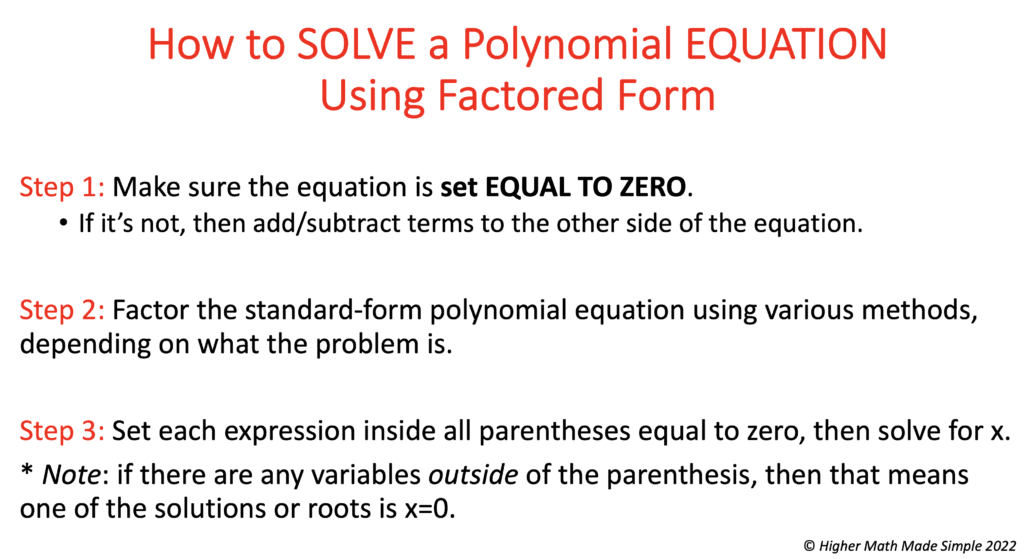
In order to solve by factoring quadratics (degree = 2), cubics (degree = 3) and more, first we must make sure that the equation is set equal to zero. After that, we then set each expression inside the parenthesis separately equal to zero. Finally we solve for x in those separate equations. ** If there is a variable outside the parenthesis (if you used the GCF method), then you must set that equal to zero too. We’ll talk more about all of this later in this blog post and with some examples.
Below are screenshots of a couple of slides from my lesson slides below:
First Things First
If you’re a teacher reading this, did you grab my *FREE* Algebra 2 Pretest (10 pages) with answers yet? Click here.
Teachers can also access my lessons, keys, reference sheets for:
If you’re a student, this topic is also one of my modules in my online course to get a solid foundation in any high school math subject, not just in Algebra 2 FYI!
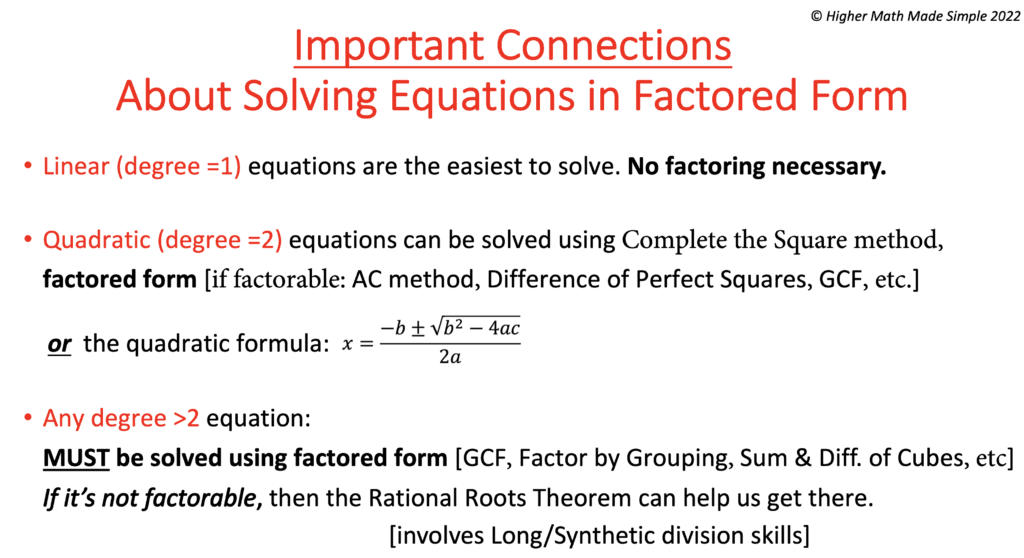
Some Clarity and Connections
Here are the 3 different ways to solve quadratic equations:
In polynomial equations where the degree (highest exponent) is larger than 2, the options are more limited:
- Rational Roots Theorem
- Factoring
We can easily see that having strong factoring skills are required as they can be used to solve all polynomial equations. Many students struggle with knowing which factoring method to use and when. Factoring allows us to break down a polynomial expression into simpler factors, which can then be used to find the roots/solutions/zeros of the equation. Remember that real roots/solutions/zeros tell us how many x-intercepts a function has.
The Importance of Setting the Equation Equal to Zero
Before we jump into the factoring methods, it’s crucial to understand that the first step before doing anything in solving polynomial equations is to make sure that the equation is set equal to zero. This is a critical foundation, since all the factoring techniques rely on the equation being in this standard form.
If the equation is not set equal to zero, then you must make it equal to zero by manipulating the equation (by adding or subtracting terms). For example: In the quadratic equation $3x^2 – 6x = 5$, we must first subtract 5 on both sides to get $3x^2 – 6x – 5 =0$.
Knowing How To Factor
If you forgot how to factor quadratics and other functions, you can refer to my YouTube video below where I cover all of the methods in depth.
The 3 Factoring Methods for Quadratic Equations
When dealing with quadratic equations (those with the degree or highest exponent of 2), there are 3 main factoring methods you should be on the lookout for:
Greatest Common Factor (GCF):
Watch my YouTube GCF Method factoring video here if you forgot how to factor polynomials using this method.
This should always be the very first thing you scan for when attempting to factor any type of polynomial. This method is where you identify the largest factor that is common to all terms in the polynomial, and factor it out.
For example: In $2x^2 + 4x=0$, both terms are divisible by 2, and they both have at least 1 x. Therefore the GCF = $2x$. When factoring using the GCF method, you always place it outside the parenthesis. On the inside of the parenthesis in this particular case should have 2 terms in it because the original polynomial has 2 terms.
The GCF method is pretty much the reverse-distributive property: $2x(x+2)=0$. If you did a “check”, performing the distributive property will get us to the original expression. Remember that the corresponding exponents should add up as this is the Product Rule. If you forgot properties of exponents, check out that blog post here.
Any time we have a factored-form equation set equal to zero, remember that in general we have to set each expression inside the parenthesis to zero, and then solve for x separately. There is only one parenthesis in this example here; we will definitely set $x+2=0$. And then at the beginning of this blog post, I stated that if there’s a variable on the outside, then we must set that equal to zero too. So $2x=0$.
Solving each separate equations, the two solutions/roots/zeros are $x=0, -2$. Since they are both real numbers, that would mean that the function has two x-intercepts at (0, 0) and (-2, 0).
AC Method: $ax^2 + bx + c = 0$ where a = 1
You can watch my AC Method factoring YouTube video where a = 1 here, or my blog post about it here.
Otherwise I’ll brush on it a little in this blog post. Again if you want the in-depth step by step scoop, then check out those resources mentioned above.
The gist of it is that standard form of a quadratic equation is $ax^2 + bx + c = 0$. I tell my students that a way to remember the AC factoring method is this statement that rhymes: Factors of $a \times c$ must add up to $ b $.
Let’s look at the example: $x^2 + 11x + 10=0$. First you should define the a, b, and c values. In this problem, a=1, b=11, and c=10. We must look at the factors $1 \times 10=10$ that add up to $b=11$ .
So the factors +1 and +10 are the lucky winners!
Now, when you are solving a=1 problems, the beauty is that there is a shortcut method. The template is $(x + \text{something})(x + \text{something})$, where the chose factors go into those “something” spots. So factored form is $(x+1)(x+10)=0$. It doesn’t matter which factor you put in first because of the commutative property of multiplication.
Again, now that we have a factored form equation set equal to zero, it’s time to set each expression inside the parenthesis to zero. Now we have $x+1=0$ and $x+10=0$. Solving those 2 equations the 2 solutions are $x=-1, -10$. Since they are both real numbers, this means that the parabola has 2 x-intercepts at (-1, 0) and (-10, 0).
AC Method: $ax^2 + bx + c = 0$ where $a \neq 1$
You can watch my AC method factoring YouTube video where $a \neg 1$ here.
For example: $2x^2 +x -6=0$. Let’s define a=2, b=1, and c=-6. Remember that Factors of $a \times c$ must add up to $ b $.
We need to find the factors of $2 \times -6=-12$ that add up to $b=1$. So the factors -3 and 4 are the lucky winners!
THE TWIST: Factor By Grouping (4 terms)
If you forgot how to use the Factor by Grouping method, you can refer to my YouTube video here about it. Or again, the blog post that I told you about earlier has that step by step method in there too.
Unfortunately in this example $2x^2 +x -6=0$, since a is not equal to 1, we can’t use the shortcut method here. We have no choice but to use the Factor by Grouping method. So what do we do with the chosen factors -3 and 4? Great question.
We’re going to split up the middle term $1x$ into 2 terms, with the chosen factors of the coefficients of those terms. In other words $1x=-3x+4x$. Note: It doesn’t matter which factor you write first because of the commutative property of addition.
So we’ll use this to rewrite $2x^2 +x -6=0$ from 3 terms to 4 terms: $2x^2 -3x+4x-6=0$. The reason we do this is because you can only use the Factor by Grouping with 4 terms. So now we have $(2x-3)(x+2)=0$.
All this work just to turn the standard form equation into factored form. Again, when we have a factored form equation that’s set equal to zero, we take each expression inside the parenthesis equal to zero, and solve separately.
So $2x-3=0$ and $x+2=0$. Solving those separately our final solutions are $x=1.5$ and $x=-2$. Since they are both real numbers, this means that the parabola has two x-intercepts at (1.5, 0) and (-2, 0).
Difference of Perfect Squares in Quadratics: $a^2 – b^2 = (a + b)(a – b)$
Unfortunately, this has nothing to do with the a or b values in standard form. A lot students confuse this, but a lot of textbooks choose the variables a and b to use as a general formula for difference of perfect squares.
Check out my in-depth blog or watch my YouTube video here if you forgot.
Otherwise I’ll give a brief overview here that to find a, you just take the square-root of the first term. To find b, you take the square-root of the second term. The template is then $(a+b)(a-b)$ where you make your substitutions.
So basically, the square-root of the first term goes in the first set of “slots”, and the square-root of the second term goes in the second set of “slots”. That’s it! Don’t make it any harder than it is.
Let’s look at $x^2 – 16 = 0$. This can be factored using the difference of perfect squares formula:
$(x + 4)(x – 4) = 0$.
Setting each expression inside the parentheses equal to zero, we find the solutions: $x= -4, 4$.
Factoring Polynomials of Degree Greater Than 2
When dealing with polynomial equations where the highest exponent is greater than 2, the factoring methods become a bit more involved. Here are the key techniques to look for:
- Greatest Common Factor (GCF): As with quadratic equations, identifying and factoring out the GCF is an important first step.
- Factoring by Grouping: If the polynomial has four terms, you can try factoring by grouping the terms into two groups and then factoring each group.
- Sum and Difference of Cubes: If the polynomial can be written as the sum or difference of two perfect cubes, you can use a specific formula to factor it.
- Difference of Perfect Squares
Example: Factoring a Degree 4 Polynomial Using GCF
Consider the equation $x^4 + 8x^3 – 9x^2 = 0$. We can start by factoring out the GCF, which in this case is $x^2$:
$x^4 + 8x^3 – 9x^2 = 0$
$x^2(x^2 + 8x – 9) = 0$
Now we can factor the remaining quadratic expression using the AC method:
$x^2(x^2 + 8x – 9) = 0$
$x^2(x + 9)(x – 1) = 0$
Setting each expression inside the parentheses equal to zero, we find the solutions: $x=0, -9, 1$.
Summary For Solve By Factoring
The key steps are:
- Ensure the equation is set equal to zero.
- Identify the factoring method(s) that apply to the specific polynomial, such as GCF, AC method, difference of perfect squares, or sum/difference of cubes.
- Factor the polynomial expression using the appropriate method(s).
- Set each expression inside the parentheses equal to zero and solve for the roots or solutions. If there is a variable outside the parenthesis, make sure to set that equal to zero too.
By mastering these steps and the various factoring techniques, you’ll be well on your way to solving a wide range of polynomial equations with confidence. Remember to practice regularly, and don’t hesitate to review the supporting videos and resources mentioned throughout this post.