In this blog post we’ll look at examples comparing the sum of cubes vs. difference of cubes formulas. This is a factoring method only for binomials (2 terms). The methods are also known as the “sum and difference of perfect cubes”, given both binomials are perfect cubes.
First Things First
If you’re a teacher reading this, did you grab my *FREE* Algebra 2 Pretest (10 pages) with answers yet? Click here.
Teachers can also access my related lessons, keys, reference sheets for:
If you’re a student, this topic is also one of my modules in my online course to get a solid foundation in any high school math subject, not just in Algebra 2 FYI!
You can watch my YouTube video where I go over examples step by step of the sum or difference of cubes method.
What is a Perfect Cube?
Before we delve into the sum and difference of perfect cubes formulas, it’s important to understand the concept of a perfect cube. A perfect cube is the result of a number multiplied by itself 3 times. In other words, if a number $x$ is multiplied by itself three times, the result is a perfect cube, which can be represented as $x^3$.
Some examples of perfect cubes include:
- $1^3 = 1$
- $2^3 = 8$
- $3^3 = 27$
- $4^3 = 64$
- $5^3 = 125$
How can we check if a number given to us in a problem is a perfect cube? Simply put, if you take the cube-root, and you get a whole number, then it’s a perfect cube! For example: 512 is a perfect cube because $\sqrt[3]{512}=8$.
Two ways that you can take the cube-root on your calculator is by:
- Raising it to the one-third power. This is the least common way.
- On a Ti-83/84 calculator, you can access the cube-root symbol by clicking the “MATH” button, and then it’s option #4.
What about examples of perfect cubes with variables in it?
The shortcut is that any perfect cube has an exponent that is divisible by 3; in other words, that are multiples of 3 (3, 6, 9, 12, …). We know this because when we take the cube-root, it gives us a whole number for an exponent. All you do is simply divide the exponent of the perfect cube by 3.
Examples of perfect cubes and reasons why:
- $x^3$ because $\sqrt[3]{x^3}=x$
- $x^6$ because $\sqrt[3]{x^6}=x^2$
- $x^9$ because $\sqrt[3]{x^9}=x^3$
- $x^{12}$ because $\sqrt[3]{x^{12}}=x^4$
Checking That The Formulas Are Correct
Below you’ll find a snapshot of my lesson slides with answer keys:
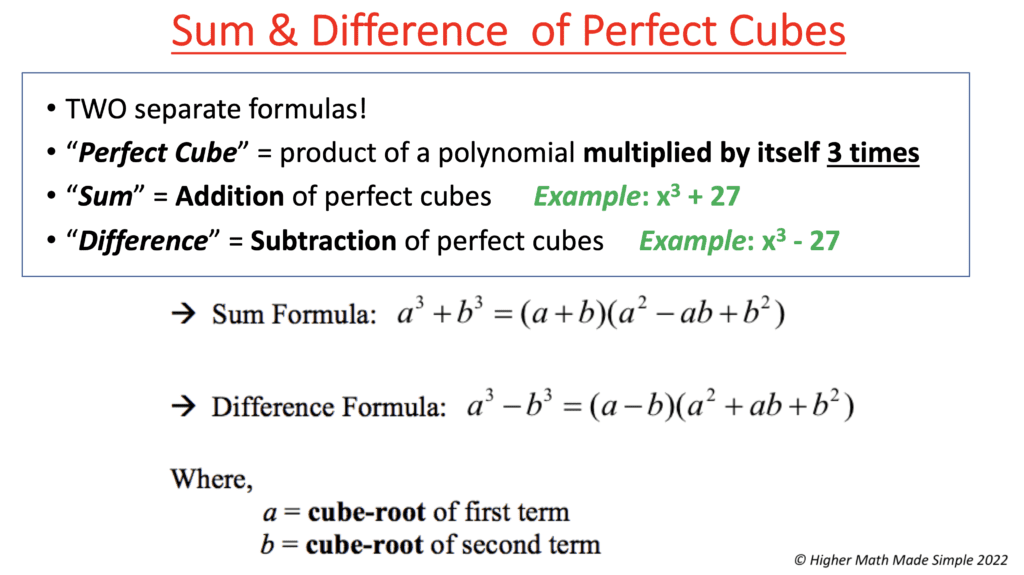
These formulas seem random to people, but they’re not just created out of thin air. Super cool short video I found on the internet is this visual representation for these formulas here.
Let’s just take one of the formulas – how about the Sum of Cubes formula – and perform the distributive property. Let’s take $(a + b)(a^2 – ab + b^2)$. If we simplify this product by the distributive property, we have: $(a^3-a^2b+ab^2)+(a^2b-ab^2+b^3)$. Notice I switched some products around because of the commutative property of multiplication.
Why would I want to do that? Because now we can combine like terms (the parenthesis aren’t actually needed). You’ll come to find that 4 terms cancel out, and then surprise-surprise! We end up with $a^2+b^2$; the sum of cubes formula.
Factoring With Sum Of Cubes Formula
The formula to factor the sum of 2 perfect cubes $a^3 + b^3$ is equal to $(a + b)(a^2 – ab + b^2)$.
In this formula, $a$ represents the cube root of the first term, and $b$ represents the cube root of the second term. The key steps to using the sum of perfect cubes formula are:
- Identify the first and second terms of the polynomial expression.
- Find the cube roots of the first and second terms, which will be $a$ and $b$, respectively.
- Substitute the values of $a$ and $b$ into the formula to factor the expression.
Sum of Cubes Example: Factor the expression $216 + 27x^3$.
Step 1: Identify the first and second terms.
The first term is 216, and the second term is $27x^3$.
Step 2: Find the cube roots of the first and second terms.
The cube root of $216$ is 6, so $a = 6$.
The cube root of $27x^3$ is $3x$ because $3x \times 3x \times 3x$, so $b = 3x$.
Step 3: Substitute the values of $a$ and $b$ into the formula $(a + b)(a^2 – ab + b^2)$.
$216 + 27x^3 = (6 + 3x)(6^2 – 6 \cdot 3x + (3x)^2)$
$216 + 27x^3 = (6 + 3x)(36 – 18x + 9x^2)$
A common mistake I see students make is that the last term they write $3x^2$ instead of $9x^2$. Remember that $(3x)$ is the entire base, so $(3x)^2=3x \times 3x=9x$.
Comparing With Difference of Perfect Cubes Formula
Both of the formulas are the same except for the signs.
The signs of the Sum of Cubes formula is + – + : $(a + b)(a^2 – ab + b^2)$
The signs of the Difference of Cubes formula is – + +: $(a – b)(a^2 + ab + b^2)$
Similar to factoring with the sum of perfect cubes formula, $a$ represents the cube root of the first term, and $b$ represents the cube root of the second term. The key steps to using the difference of perfect cubes formula are:
- Identify the first and second terms of the polynomial expression.
- Find the cube roots of the first and second terms, which will be `a` and `b`, respectively.
- Substitute the values of $a$ and $b$ into the formula to factor the expression.
Difference of Cubes Example: Factor the expression $8x^3 – 64$.
Step 1: Identify the first and second terms.
The first term is $8x^3$, and the second term is $64$.
** Technically speaking, the 64 term is negative when we read it as a binomial. However we will leave it positive since the “minus” sign tells us that we are plugging it into the difference of cubes formula template. Many students get confused with this, so I figured I’d point that out here.
Step 2: Find the cube roots of the first and second terms.
The cube root of $8x^3$ is $2x$, so $a = 2x$.
The cube root of $64$ is $4$, so $b = 4$.
Step 3: Substitute the values of $a$ and $b$ into the formula.
$8x^3 – 64 = (2x – 4)((2x)^2 + 2x \cdot 4 + 4^2)$
$8x^3 – 64 = (2x – 4)(4x^2 + 8x + 16)$
Be careful to write $4x^2$ instead of $2x^2$ (common mistake). This is because $(2x)$ is the entire base of the exponent. So $(2x)^2=2x \times 2x=4x^2$.